
What is Hexagonal Thinking?
Hexagonal thinking involves students recording ideas, questions or facts on hexagonal tiles and arranging them so that related tiles are next to each other, essentially building a web of connections.
It can be used to provide students with an effective way to launch project-based-learning, and students can go after interesting questions.
I will show you how to use the strategy within a rigorous project-based-learning (PBL) methodology.
Rigorous PBL

Michael McDowell, author of “A Framework for Fostering Rigor in PBL”, defines rigor as the equal intensity and integration of the following levels of learning:
- surface (I know ideas or skills),
- deep (I can relate ideas and/or skills), and
- transfer (I can apply ideas or skills in multiple contexts).
McDowell explains that rigorous PBL is an inquiry-based methodology that follows a distinct pathway of learning. It organizes levels of rigor into a particular sequence. McDowell notes that “students experience all levels of complexity and the need to learn each level to accomplish a challenge task.” McDowell calls this entry event where students encounter a challenging task and build the need for surface and deep learning.
McDowell states, “The process integrates all levels of learning through six steps.” One way to do this is through hexagonal thinking.
The Six Steps of Hexagonal Thinking
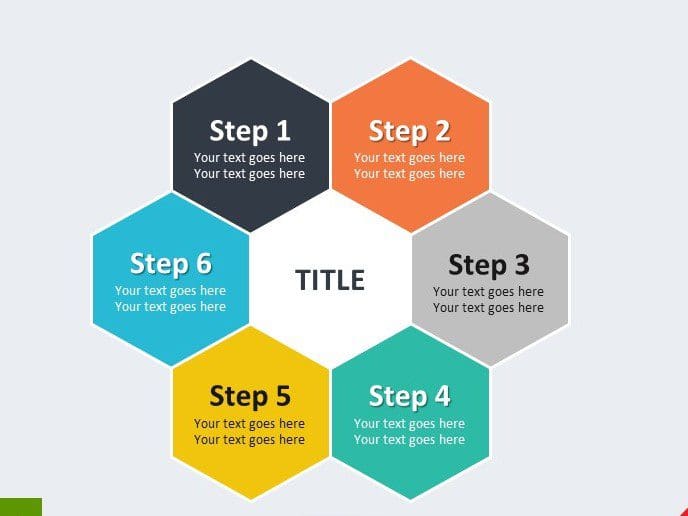
McDowell lists the 6 steps below:
Step 1: Launch. Provide a challenging context (or set of contexts) to initiate intellectual engagement for students. For instance, you may have students listen to the Revisionist History podcast on college rankings or view the introductory video of bringing back the woolly mammoth.
Step 2: Surface exploration. Provide students with a set of hexagonal shapes (e.g., a set of hexagonal sticky notes or a free electronic version) that enable them to write down key terms that are important. Share with students that one color should represent contexts (situations in which the content applies) and another color should represent the content (specific knowledge or skills that students must learn and apply to other situations). One scaffold that may be helpful to start this process is to provide students with a word bank.
Step 3: Deep-level connections. This step is conducted in two parts or sub-steps. First, ask students to find connections between the hexagons they have labeled. Here, students should discuss (or write down) the connections between content, between contexts, and across both content and contexts. Next, have students compare and contrast their hexagonal structures and rationale for each connection with other students (or student groups).
Step 4: Transfer-level extensions. Ask students to generate additional contexts and/or content to consider in this project. This is an opportunity to prompt students to
- create analogies,
- Compare contexts within and across groups,
- generate hypotheses on how their configurations will change over time, and
- draft questions they want to solve.
Step 5: Driving questions and learning goals. Have students share their thinking from step four with others to identify the driving question, learning intentions, and success criteria. One way to do this is to use structured protocols (e.g., chat stations).
Perspectives (Chat 1): What are potential perspectives that emerge from this problem? What is your current “position” on this problem? What else would you want to know about this situation?
Problem (Chat 2): What do you see as potential driving questions from the connections made between different groups?
Potential Outcomes (Chat 3): What do you think we will need to learn to address these questions and understand the connections more deeply?
Patterns and Potential Connections (Chat 4): What other contexts can you think of that connect to this work? Do you see any recurring patterns?
Step 6: Develop knows/needs to know. During this final step, students and teachers determine which driving question or set of questions they will work to answer. As part of answering this question, teachers provide a rubric and ask students to compare and contrast their thinking of what they are learning with the teacher.
McDowell believes that “This is an opportunity for students to write down what they “know” from the rubric, as well as what they “need to know” to meet the expectations on the rubric and address the driving question. Students then draft any next steps they will take to answer the driving question(s) and meet the learning intention(s) and success criteria. Finally, students generate hypotheses on how their hexagonal configurations will change over time.”
Examples of hexagonal thinking in the classroom
Betsy Potash, author of “What is Hexagonal Thinking? Plus 13 Examples of to Inspire You”, provides examples of hexagonal thinking in the classroom:

7th grade students of Genevieve Chavez, Spain

Students of Ruth Anne Flowers, Airport High School, South Carolina

Students of Tabatha Rose, Strath Haven High School, Pennsylvania
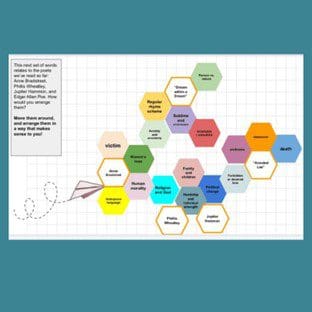
Students of Megan Elizabeth
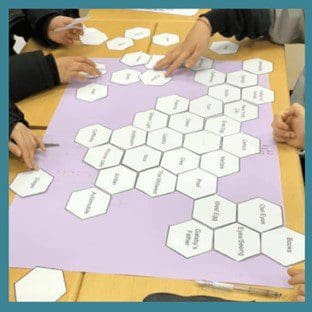
Students of Sharon Ma, Shanghai SMIC Private School, China
